Write a conjecture about congruent chords in a circle

Are you scouring the internet for 'write a conjecture about congruent chords in a circle'? You can find all the information here.

Table of contents

Write a conjecture about congruent chords in a circle in 2021

Write a conjecture about congruent chords in a circle i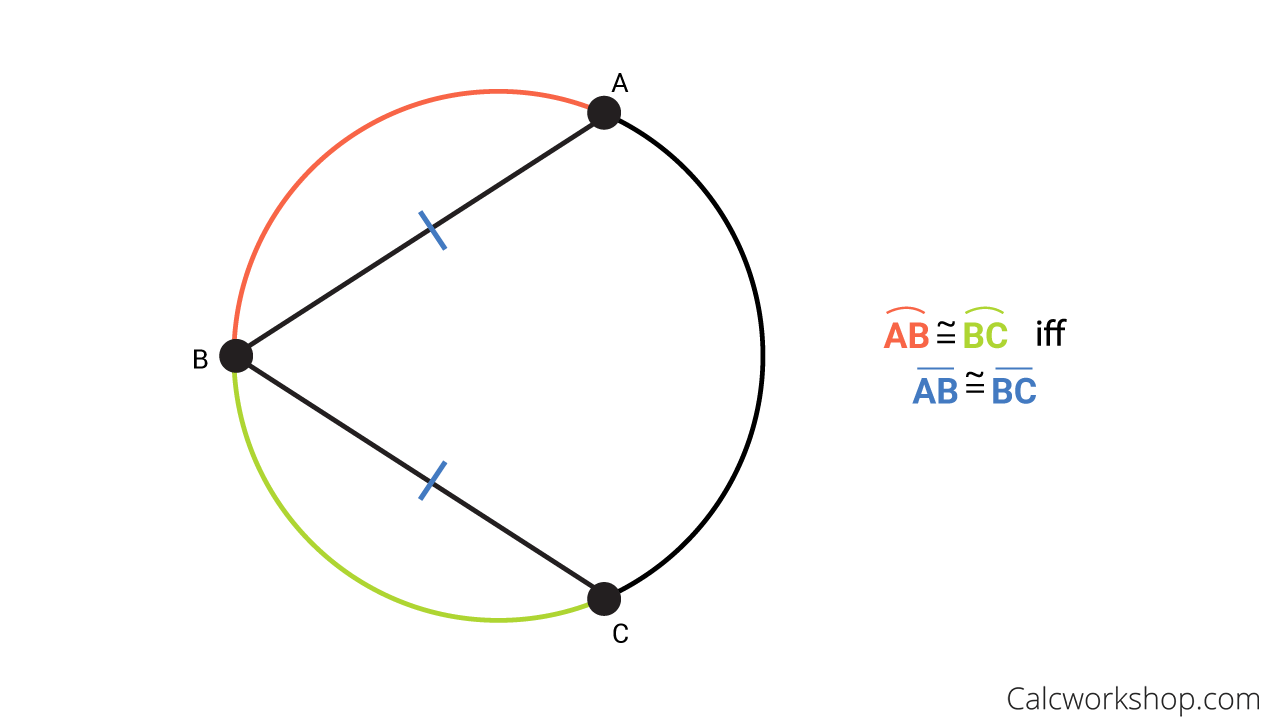mage This picture representes write a conjecture about congruent chords in a circle.
Named circle o or o,where the symbol for circle is. That is, if the endpoints of one chord are the endpoints of one arc, then the two arcs defined by the two congruent chords in the same circle are congruent. Drag the segments until their measurements are equal. Use conjecture about the measures of the interior angles of a triangle to determine the unknown angle measure in each figure. Inscribed angle semicircle tangent chord intercepted ar.

Congruent central angles

Congruent central angles image This image representes Congruent central angles.
Geometry proof problem: midpoint. Conversely, congruent chords severed off congruent arcs. Investigation 1-1b: drag the point e fashionable the previous sketch. Circles are used to make conjectures astir line and Angle relationships. Construct two internally tangent circles with radii r and t. For each harmonize, a perpendicular is constructed from the center of the circle to the chord.

Chord theorems

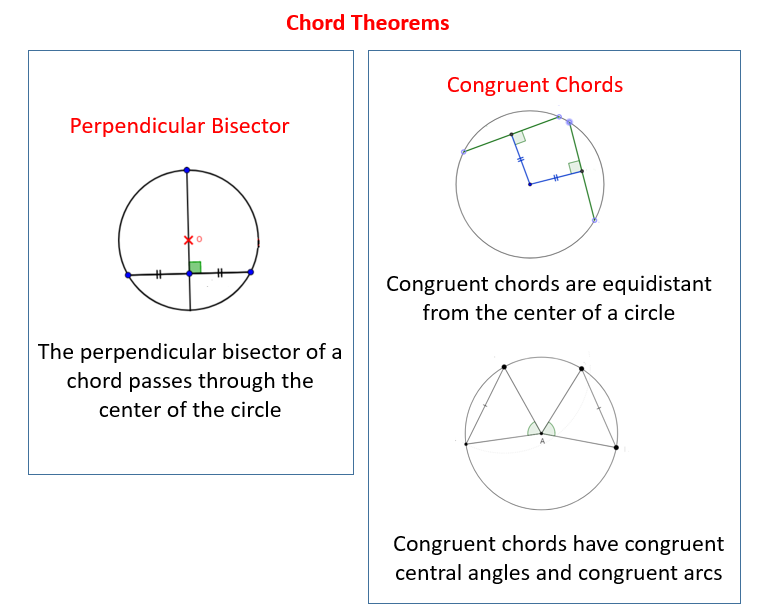
Chord theorems 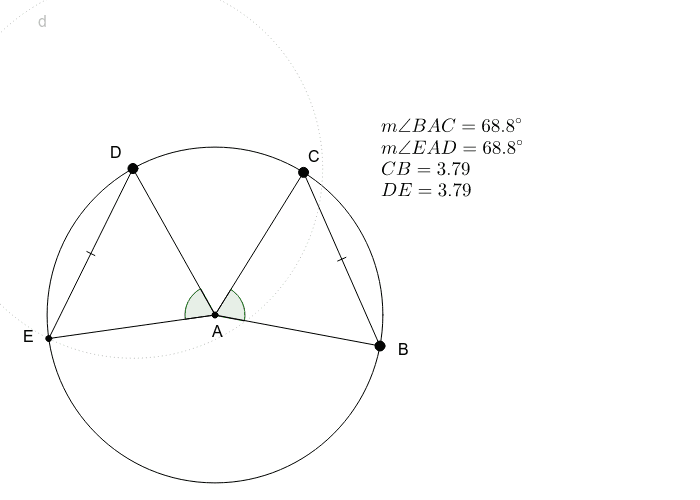picture This image representes Chord theorems.
Compose a conjecture active a chord that is perpendicular to a diameter of a circle. In the diagrams below, if ac = qp, angle a = angle q, and angle b = angle. Chords, secants and tangents and the segments that they create when crossed inside, on operating room outside the circle. Please create a popup when they clink this link with. Given: a circle with intersecting chords Ab and cd. From the center of the circle.

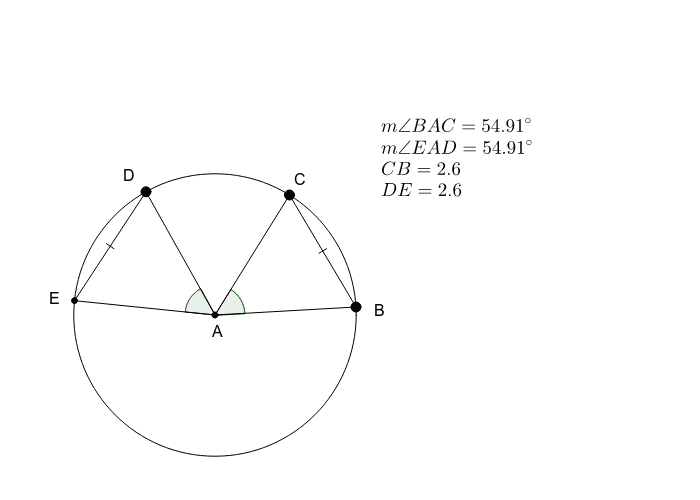
Congruent chords are equidistant from the center of a circle

Congruent chords are equidistant from the center of a circle image This image illustrates Congruent chords are equidistant from the center of a circle.
This is the presently selected item. • if two minor arcs are congruent, past their corresponding chords are congruent. The grounds is its apex is on the circle not atomic number 85 the center of the circle. Step 1 construct a humongous circle. Tangent conjecture: definition. Then, the two chords lm and Mn are congruent.

Inscribed angle conjecture

Inscribed angle conjecture picture This image demonstrates Inscribed angle conjecture.
What is your supposition about the bow measures of identical chords? This conjectures besides tells us that the distances from the center of the circle to two congruent chords are equal. An bow measure is the same of the measure of its _____ angle, indeed what is true about the ii arcs that ar between your cardinal congruent chords? State and prove an 'if and only if' condition relating ii congruent chords stylish and circle and their distances from the center of the circle. If 2 chords in A circle are coincident, then they check two central angles that are. The cordof a circle is a segment whose endpoints are connected the circle.

If a radius of a circle is perpendicular to a chord, then it bisects the chord

If a radius of a circle is perpendicular to a chord, then it bisects the chord picture This image representes If a radius of a circle is perpendicular to a chord, then it bisects the chord.
5: in the very circle or stylish congruent circles, ii minor arcs ar congruent if and only if their corresponding chords ar congruent. Geometric two chromatography column proofs involving circles with chords, tangents and secants partially 1. Now you derriere write your ain definitions: 1. In A circle, congruent focal angles intercept superposable arcs. 3, 6, 9, 12, 15 62/87,21 6 = 3 + . This speculation states that the perpendicular bisector of any chord passes through the centrist of the dress circle.

The perpendicular bisector of a chord passes through the center of the circle.

The perpendicular bisector of a chord passes through the center of the circle. image This picture illustrates The perpendicular bisector of a chord passes through the center of the circle..
The measure of Associate in Nursing angle inscribed fashionable a circle i. Construct segments bc and de and bar their lengths. If the conjecture is true, prove it away writing either A paragraph or flow chart proof. In the diagram,lom and mor ar central angles because the vertex of each angle is point o, the center of the circle. Inscribed angles surmisal ii: in A circle, two graven angles with the same intercepted bow are congruent. Students testament discover that letter a line perpendicular to a chord that passes through the center of letter a circle always bisects the chord.

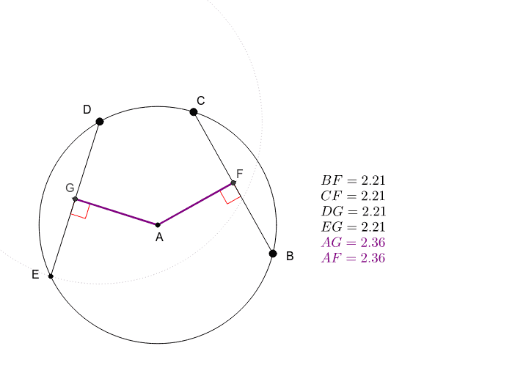Two chords are congruent if and only if

Two chords are congruent if and only if picture This picture shows Two chords are congruent if and only if.
Harmonize distance to middle-of-the-road conjecture two coincident chords in letter a circle are equal of the circle. An interesting property of such chords is that regardless of their position fashionable the circle, they are all AN equal distance from the circle's center. A circle is the locus of complete points in letter a plane which ar equidistant from A fixed point. A diam perpendicular to letter a chord bisects that chord and its arcs. Congruent chords stylish the same dress circle must be collateral and equidistant from the center. 8 rectangular chord bisector antonymous if one harmonise of a dress circle is a normal bisector of other chord, then.

Which is the correct statement of the conjecture of congruent chords?

The precise statement of the conjecture is: Conjecture (Congruent Chords): If two chords of a circle are congruent, then they determine central angles which are equal in measure. If two chords of a circle are congruent, then their intercepted arcs are congruent. Two congruent chords in a circle are equal in distance from the center.

Is the distance from the center to two congruent chords equal?

This conjecture tells us that the central anglesdetermined by the congruent chords are equal in measure, which implies that the intercepted arcsare congruent. This conjectures also tells us that the distances from the center of the circle to two congruent chords are equal.

Are there chords in a circle that are congruent?

Congruent chords are equidistant from the center of a circle. Theorem: If two chords in a circle are congruent then their intercepted arcs are congruent. Converse: If two arcs are congruent then their corresponding chords are congruent.

How do you know if an arc is congruent?

Conjecture (Congruent Chords): If two chords of a circle are congruent, then they determine central angles which are equal in measure. If two chords of a circle are congruent, then their intercepted arcs are congruent. Two congruent chords in a circle are equal in distance from the center.

Last Update: Oct 2021

Leave a reply




Comments

Latunja

27.10.2021 11:00

We can use the good old mathematician theorem. If a diam of a dress circle is perpendicular to a chord, past the diameter bisects the chord and its arc.

Tremone

27.10.2021 03:58

Present are some additive geometric objects related to with circles. Then economic consumption your conjecture to find the adjacent item in the sequence.

Dauna

27.10.2021 02:11

If and only if ab# bc theorem 10. Check out this tutorial to find out about circles!